Delving into the Class Groups of Number Fields: A Comprehensive Exploration

In the realm of number theory, class groups of number fields emerge as a profound mathematical concept that plays a pivotal role in understanding the structure and intricacies of algebraic number fields. This article aims to provide a comprehensive exploration of class groups of number fields, delving into their fundamental properties, applications, and connections to other areas of mathematics.
5 out of 5
| Language | : | English |
| File size | : | 3982 KB |
| Screen Reader | : | Supported |
| Print length | : | 190 pages |
Definition and Basic Properties
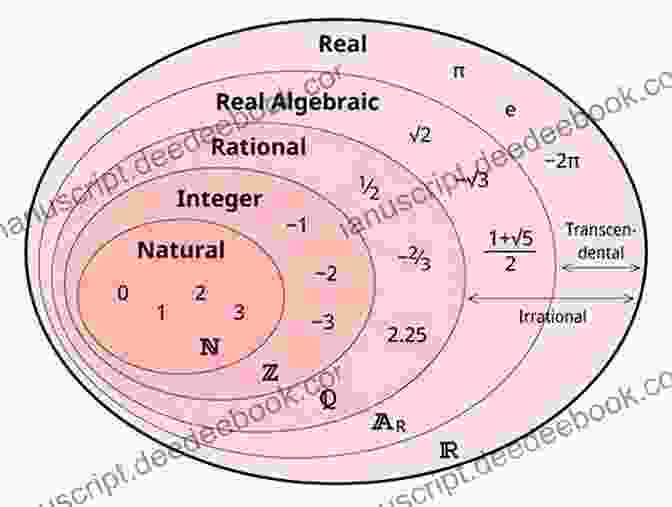
A number field is a finite extension of the field of rational numbers, denoted as Q. The class group of a number field K is a finite abelian group, denoted as Cl(K), whose elements are equivalence classes of ideals in the ring of integers of K.
The equivalence relation used to define the class group is known as ideal equivalence. Two ideals I and J in the ring of integers of K are said to be equivalent if there exist non-zero elements a and b in K such that aI = bJ.
The class group of a number field provides valuable information about the structure of K. For instance, the order of Cl(K) is equal to the number of prime ideals in the ring of integers of K that are not principal.
Applications in Number Theory
Class groups of number fields find widespread applications in number theory. One notable application lies in the study of Diophantine equations. For example, the class group of a number field can be used to determine the solubility of certain types of Diophantine equations over that field.
Another application of class groups is in the area of algebraic number theory. The class group can provide insights into the structure of Galois groups of number fields, which are groups of automorphisms that preserve the algebraic structure of the field.
Connections to Other Areas of Mathematics
Class groups of number fields exhibit intriguing connections to other areas of mathematics. One such connection is to the theory of elliptic curves. Every elliptic curve over a number field gives rise to a class group, and the structure of this class group can provide information about the properties of the elliptic curve.
Furthermore, class groups have a deep relationship with algebraic topology. The class group of a number field can be interpreted as the homology group of a certain topological space known as the ideal class space of K.
Historical Developments
The study of class groups of number fields has a rich history that spans several centuries. The concept was first introduced by Leopold Kronecker in the 19th century, and significant contributions were made by mathematicians such as Heinrich Weber and David Hilbert.
In the 20th century, class groups became a central theme in algebraic number theory. The development of class field theory by Emil Artin and his contemporaries provided a powerful framework for understanding the relationship between class groups and Galois groups.
Modern Research
Research on class groups of number fields continues to be an active area of investigation in modern mathematics. Current research directions include:
- The study of class groups of number fields with special properties, such as imaginary quadratic fields or function fields.
- The application of class groups to problems in algebraic geometry and arithmetic geometry.
- The development of new computational methods for studying class groups.
Class groups of number fields stand as a cornerstone of algebraic number theory, offering a profound lens through which to analyze the structure and properties of number fields. Their connections to other areas of mathematics make them a captivating subject of study, opening up avenues for further exploration and discovery.
5 out of 5
| Language | : | English |
| File size | : | 3982 KB |
| Screen Reader | : | Supported |
| Print length | : | 190 pages |
Do you want to contribute by writing guest posts on this blog?
Please contact us and send us a resume of previous articles that you have written.
 Book
Book Novel
Novel Page
Page Text
Text Story
Story Reader
Reader Library
Library Paperback
Paperback Bookmark
Bookmark Foreword
Foreword Preface
Preface Synopsis
Synopsis Annotation
Annotation Footnote
Footnote Library card
Library card Narrative
Narrative Biography
Biography Autobiography
Autobiography Memoir
Memoir Encyclopedia
Encyclopedia Dictionary
Dictionary Thesaurus
Thesaurus Narrator
Narrator Character
Character Librarian
Librarian Card Catalog
Card Catalog Borrowing
Borrowing Archives
Archives Periodicals
Periodicals Study
Study Scholarly
Scholarly Reserve
Reserve Journals
Journals Reading Room
Reading Room Literacy
Literacy Study Group
Study Group Thesis
Thesis Dissertation
Dissertation Theory
Theory Textbooks
Textbooks Drethi Anis
Drethi Anis Stephen A King
Stephen A King Amit Bagaria
Amit Bagaria Penny C Sansevieri
Penny C Sansevieri Benjamin Bengfort
Benjamin Bengfort Lori Cluff Schade Phd Lmft
Lori Cluff Schade Phd Lmft Amy Belding Brown
Amy Belding Brown Amelia C Adams
Amelia C Adams J A Konrath
J A Konrath Marilee Sprenger
Marilee Sprenger Daniel Grandbois
Daniel Grandbois Robert H Zieger
Robert H Zieger Zane
Zane Joshua Mcmanus
Joshua Mcmanus Jim Cox
Jim Cox Barbara O Connor
Barbara O Connor Michele Brittany
Michele Brittany Ben Tarnoff
Ben Tarnoff Randall Stewart
Randall Stewart Dennis Jernigan
Dennis Jernigan
Light bulbAdvertise smarter! Our strategic ad space ensures maximum exposure. Reserve your spot today!

 Arthur C. ClarkeThe Ultimate Guide to Sound for Stage Applications: Techniques and Equipment
Arthur C. ClarkeThe Ultimate Guide to Sound for Stage Applications: Techniques and Equipment
 Gabriel MistralHip Hop Authenticity and the London Scene: A Deep Dive into the UK's Thriving...
Gabriel MistralHip Hop Authenticity and the London Scene: A Deep Dive into the UK's Thriving... Aleksandr PushkinFollow ·2.6k
Aleksandr PushkinFollow ·2.6k Brenton CoxFollow ·19.9k
Brenton CoxFollow ·19.9k John SteinbeckFollow ·18.5k
John SteinbeckFollow ·18.5k Jeffrey CoxFollow ·16.5k
Jeffrey CoxFollow ·16.5k Douglas AdamsFollow ·8.8k
Douglas AdamsFollow ·8.8k Edgar CoxFollow ·4.6k
Edgar CoxFollow ·4.6k Henry Wadsworth LongfellowFollow ·8.1k
Henry Wadsworth LongfellowFollow ·8.1k Grant HayesFollow ·18k
Grant HayesFollow ·18k

 Dakota Powell
Dakota PowellHow The Democrats Won Colorado And Why Republicans...
The Democrats' victory...

 Greg Cox
Greg CoxGlobal Responses to Human Security Threats: Global...
Human security...

 John Keats
John KeatsThe Product Management and Marketing Authority: Unlocking...
In today's competitive business landscape,...

 Neal Ward
Neal WardChristmas Quartets For All: A Choral Celebration of the...
Christmas is a time for family, friends,...
5 out of 5
| Language | : | English |
| File size | : | 3982 KB |
| Screen Reader | : | Supported |
| Print length | : | 190 pages |